Heat transfer in agitated vessels can be carried out either through an external jacket on the vessel or by internal coils. Where a jacket or coils cannot provide the surface area required, a recirculation loop with an external heat exchanger may be used. In this case the heat exchanger would be designed by the normal methods and will not be covered further in this chapter. (See Heat Exchangers.)
A jacket may be either a full conventional jacket, a dimpled jacket, or a half-pipe jacket, often called a limpet coil, as illustrated in Figure 1a, Figure 1b and Figure 1c. The designs are compared by Markovitz (1971).
A conventional jacket has the advantage that it covers the full wall and base surface and is very simple to construct. A dimpled jacket allows construction from light gauge metals while maintaining strength. A half-pipe jacket may be cheaper for a high pressure on the service side and has the advantage that more than one service can be supplied to different sections of the wall. However, a limited amount of the surface will be covered by a half-pipe jacket, the large amount of weld can cause mechanical concerns where thermal cycling occurs and the jacket welding must be spaced from the dished end main welds to maintain mechanical integrity of the vessel wall.
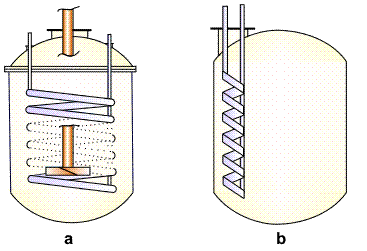
Internal coils may be full helical coils, or a number of smaller, ringlet coils. Figure 2a and b.
A full helical coil is the more usual design, allowing the maximum surface to be installed, but requires a two-piece vessel with a relatively expensive main flange. Smaller ringlet coils can be designed to be inserted through large branches on the upper vessel dished end, but can leave quiescent, unmixed regions within their circumference.
The choice between a jacket and coils is based on a number of considerations. For highly corrosive or highly reactive materials, a jacket has the advantage that there are no extra materials of construction and no extra metal surface in contact with the process other than the normal vessel wall. There is also less risk of cooling fluid coming into contact with the reaction mass. For the manufacture of pharmaceuticals, fine chemicals and performance products, a jacket minimizes contamination as there are no extra surfaces to clean. For materials with difficult rheology the full range of agitator designs can be used with a jacket without difficulty. However, a jacket has a lower heat transfer performance than a coil as there will be a lower process side coefficient, usually a greater wall thickness, and a smaller surface area. A jacket may also require a higher service side flow. For exothermic reactions, a jacketed vessel has the disadvantage that the area/volume ratio decreases with increasing scale. The use of a greater height/diameter ratio at larger scale can help to reduce this problem, but only to a limited extent. A coil has the advantage that a large surface area can be provided, for example, in one particular highly exothermic reaction 18 m2m−3 has been installed in a 5 m3 reactor. However, it is important not to pack the coil so tightly as to form a false wall.
Agitated vessel heat transfer is commonly used in batch manufacture where it is frequently necessary to calculate the time to heat or cool a batch or the cooling capacity required to hold an exothermic or endothermic reaction at constant temperature. It may also be necessary to define the stable operating region or acceptable reagent addition rate for an addition controlled highly exothermic semi-batch reaction. The heat removal rate is defined by:

For the simple case of the time to cool or heat a batch of mass, M:

For a constant service side temperature, Ts, for example, steam heating:

The time to reach a temperature, T from a starting temperature Tt=0 is:

For more complex situations numerical integration may be required, but there are many suitable dynamic simulation languages available. For coils and jackets, the Overall Heat Transfer Coefficient can be calculated in the usual way:

where α and αs are the process and service side heat transfer coefficients, respectively. The service side fouling resistance, l/αf, will be available from local experience or from Kern (1950) , for example. As a general guide, approximate overall coefficients typical of agitated jacketed vessels are given in Tables 1 and 2.
Table 1. Typical overall coefficients for jacketed glass lined steel vessels
| Duty | U (W m−2K−1) |
| Distillation/Evaporation | 350 |
| Heating | 310 |
| Cooling | 200 |
| Cooling (chilled service) | 100 |
Table 2. Typical overall coefficients for jacketed carbon and stainless steel vessels
| Duty | U (W m−2K−1) |
| Heating | 400 |
| Cooling | 350 |
| Cooling (chilled service) | 150 |
A typical overall coefficient for a well designed coil would be 400 to 600 Wm2K−1.
Under normal circumstances the overall coefficient should be dominated by the process side. However, for the service side the following guidelines should be observed to ensure good performance:
For jackets:
A conventional jacket should be fitted with baffles (see Figure 1a).
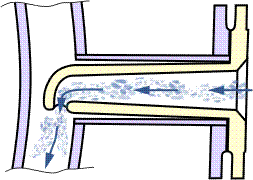
Service injection nozzles should be used to direct the service flow, especially for a glass-lined steel vessel (see Figure 3).
A vent should be fitted and maintained to prevent gas blanketing.
For a plain jacket with liquid service the target circumferential velocity should be 1-1.5 ms−1.
For a half-pipe jacket with liquid service the minimum target velocity should be 2.3 ms−1.
For a dimpled jacket with liquid service, pressure drop may limit the velocity to 0.6 ms−1.
For an internal coil:
For a liquid service, the minimum target velocity should be 1.5 ms−1.
There are several correlations available for the service side coefficient, depending on the jacket or coil design and the flow regime of the service fluid. Fletcher (1987) provides some useful guidance and the research club, HTFS has also produced a design report for members. The following correlations are recommended:
Conventional unbaffled jacket, liquid service with high flow [(Lehrer (1970)]
(6)
where:
(7)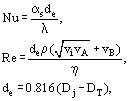
vi is the velocity at the inlet nozzle or branch and vB is the velocity component due to buoyancy, Dj is the jacket diameter and DT is the vessel diameter.
(8)
where
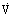 is the volumetric flow rate, di is the diameter of the inlet, z is the wetted height of the jacket, β is the fluid thermal expansion coefficient, and ΔTs is the temperature rise of the service fluid. VA is the rise velocity in the jacket annulus and depends on the inlet orientation:
(9)
is the volumetric flow rate, di is the diameter of the inlet, z is the wetted height of the jacket, β is the fluid thermal expansion coefficient, and ΔTs is the temperature rise of the service fluid. VA is the rise velocity in the jacket annulus and depends on the inlet orientation:
(9)
Conventional unbaffled jacket, liquid service with high flow [(Lehrer (1970)] (see also Free Convection)
(10)
Here,
K = 0.15 for upward flow, heating; downward flow cooling; K = 0.128 for downward flow heating; upward flow cooling; ΔTm is the mean temperature difference between the service and the vessel wall (11)
Baffled or dimpled jacket, liquid service. The service side coefficient for a baffled or dimpled jacket will be greater than for an unbaffled jacket with high flow, therefore the above can be used as a conservative estimate. Using the correlation for a half-pipe coil with the flow area equivalent to the baffle channel is not recommended as it may give an overestimate.
Half-pipe coil, liquid service
(12)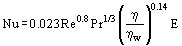
This is the normal Sieder-Tate equation, (see Forced Convective Heat Transfer) applied to the whole jacketed area with the effectiveness factor (E) from Kneale (1969), typically 0.8–1. Nu and Re are based on the hydraulic mean diameter, de:
(13)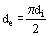
Condensing service. A condensing coefficient in a jacket should be extremely high compared to the process side and normally αs−1 ~ 0. A conservative estimate can be obtained from the Nusselt analysis [see Kern (1950)]:
(14)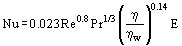
The physical properties refer to the liquid phase and ΔT = Tsat - Twall (see Condensation).
The process side coefficient will be determined by the agitator type and speed. (See also Agitation Devices.) For low viscosity fluids, most turbine-type high-speed agitators can give good performance. For high viscosity and Non-Newtonian Fluids, larger diameter agitators will be required. Harnby et al. (1985) and Oldshue (1983) provide guidance on agitator selection. For Non-Newtonian Fluids, there are two important considerations:
A mean apparent viscosity is required to calculate Nu from Re. The normal practice is to estimate it using the Metzner-Otto approach, where:
(15)
Kmo depends on the agitator type, N is the rotational speed (s -1).
The viscosity correction only allows for the effect of temperature near the wall and NOT the distribution of shear rate. It will normally be sufficient to assume an homogeneous distribution of shear rate. Calculation of a wall shear rate to predict a local ηa is beyond the scope of this article.
It is essential to ensure that a material with a significant yield stress is maintained fluidized right up to the vessel wall. Nienow (1988) reviews the design techniques. Use the vessel diameter (DT) as the diameter of the region to be fluidized:
(16)
Po is the impeller Power number [Harnby et al. (1985)], and:
(17)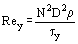
For the vessel wall surface, the process side coefficient can be calculated from:
(18) (19)
(19)
For transfer to an internal coil:
(20)
The conductivity of the wall material can be found in standard texts [Kern, (1950)]. The resistance may be significant for some vessels linings, for example, glass lined steel, where the manufacturer's data should be consulted. There will also be some limitations on the ability of glass lining to withstand thermal shock.
REFERENCES
Barton, E. and Williams, E. V. (1950) Experimental Determination of Film Heat Transfer Coefficients, Trans. I. Chem. E., 17:3.
Fletcher, P. (1987) Heat Transfer Coefficients for Stirred Batch Reactor Design, The Chemical Engineer, April.
Harnby, N., Edwards, M. F., and Nienow A. W. (1985) Mixing in the Process Industries, Butterworths.
Kern, D. Q. (1950) Process Heat Transfer, New York: McGraw-Hill.
Kneale, M. (1969) Design of Vessels with Half Coils, Trans. I. Chem. E., 47.
Lehrer, I. H. (1970) Jacket-side Nusselt Number, Ind. Eng. Chem. PDD, 9:4.
Markovitz, R. E. (1971) Picking the Best Vessel Jacket, Chemical Engineering, Nov. 15th.
Nienow, A. W. (1988) Aspects of Mixing in Rheologically Complex Fluids, Chem. Eng. Res. Des. 66:1.
Oldshue, J. Y. (1983) Fluid Mixing Technology, New York: McGraw-Hill.
Использованная литература
- Barton, E. and Williams, E. V. (1950) Experimental Determination of Film Heat Transfer Coefficients, Trans. I. Chem. E., 17:3.
- Fletcher, P. (1987) Heat Transfer Coefficients for Stirred Batch Reactor Design, The Chemical Engineer, April.
- Harnby, N., Edwards, M. F., and Nienow A. W. (1985) Mixing in the Process Industries, Butterworths.
- Kern, D. Q. (1950) Process Heat Transfer, New York: McGraw-Hill.
- Kneale, M. (1969) Design of Vessels with Half Coils, Trans. I. Chem. E., 47.
- Lehrer, I. H. (1970) Jacket-side Nusselt Number, Ind. Eng. Chem. PDD, 9:4.
- Markovitz, R. E. (1971) Picking the Best Vessel Jacket, Chemical Engineering, Nov. 15th.
- Nienow, A. W. (1988) Aspects of Mixing in Rheologically Complex Fluids, Chem. Eng. Res. Des. 66:1.
- Oldshue, J. Y. (1983) Fluid Mixing Technology, New York: McGraw-Hill.