Radiation of Isothermal Volumes of Scattering Medium: An Error of the Diffusion Model
Following from: An estimate of P1approximation error for optically inhomogeneous media; Diffusion approximation in multidimensional radiative transfer problems
Leading to: Finite-element method for radiation diffusion in nonisothermal and nonhomogeneous media; Radiative transfer in multidimensional problems: A combined computational model
Following the early paper by Dombrovsky and Barkova (1986), we consider analytical solutions for thermal radiation of a homogeneous scattering medium in an infinite channel of rectangular cross section and in a finite cylinder. It is assumed that the medium has constant temperature T and channel walls have equal temperatures Tw.
For the rectangular channel, we chose a coordinate system in a channel cross section with axes x and y parallel to the walls and with the coordinate origin in the geometrical center of the cross section, so that channel walls will have coordinates ∓ x0 and ∓ y0. Solving the boundary-value problem
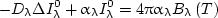
|
(1a) |

|
(1b) |
in the region 0 ≤(x, y) ≤(x0, y0) with the use of separation of variables, we obtain the following expressions for the spectral radiation flux toward the wall parallel to the x axis and for the corresponding spectral emissivity:
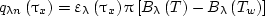
|
(2a) |
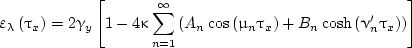
|
(2b) |

|
(2c) |

|
(2d) |
where (τx, τy, τx0, τy0) = βλtr · (x, y, x0, y0); γx = γw(εwx), γy = γw(εwx); εwx, εwy are the emissivities of the walls at x = ∓ x0 and y = ∓ y0, respectively; κ = 2 - Nappr/ 2; and μn,νn,μ'n,ν'n are the characteristic values of the problem:

|
(3a) |

|
(3b) |
Let us consider a similar problem for a cylinder of radius r0 and length 2x0. The origin of cylindrical coordinates is placed at the axis of the cylinder at the point with equal distances from the ends. The analytical solution for the emissivity variation along the cylindrical surface ελ(τx) = E(τx, τr0) and along the bottom surface ελ(τr) = E(τx0, τr) is as follows:

|
(4a) |

|
(4b) |

|
(4c) |
The characteristic values μn,νn,μ''n,ν''n are determined from the transcendental equations

|
(5a) |
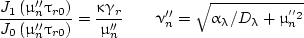
|
(5b) |
For solving the transcendental equations for characteristic values, it is necessary first to find zero and discontinuous point of functions on the left side of these equations. We have used the McMagon expansion for zero points of the Bessel functions J0 and J1 (Abramowitz and Stegun, 1965). The subsequent iterations of characteristic values provide the values with relative error less than 10-5. Since summation of series (2) and (4) is a difficult task, we have considered the dependence of the computational error on the number of the series terms with Nser taken into account. An example of such a calculation of the spectral emissivity of nonscattering medium in a square channel at Nappr = 0 is presented in Table 1. One can see that the number of series terms that should be taken into account increases with the optical thickness of the medium. Calculations have shown that Nser can be chosen according to the formula

|
(6) |
As soon as the two-dimensional problem at max{τx0, τy0} > 5 is degenerated, it is sufficient to use analytical solutions with no more than 50 characteristic functions for all the substantially two-dimensional situations.
Table 1. Convergence of analytical solution for emissivity of a nonscattering medium in a square channel with the number of series terms taken into account
| x/ x0 | ε | |||||
| τ0 = 1 | τ0 = 5 | |||||
| Nser = 10 | 30 | 50 | Nser = 10 | 30 | 50 | |
| 0 | 0.897 | 0.897 | 0.897 | 1.005 | 1.000 | 1.000 |
| 0.1 | 0.894 | 0.895 | 0.895 | 0.995 | 1.000 | 1.000 |
| 0.2 | 0.888 | 0.888 | 0.888 | 1.005 | 1.000 | 1.000 |
| 0.3 | 0.876 | 0.876 | 0.876 | 0.995 | 1.000 | 1.000 |
| 0.4 | 0.858 | 0.858 | 0.858 | 1.005 | 1.000 | 0.999 |
| 0.5 | 0.834 | 0.834 | 0.834 | 0.992 | 0.998 | 0.998 |
| 0.6 | 0.802 | 0.801 | 0.801 | 1.001 | 0.995 | 0.995 |
| 0.7 | 0.758 | 0.758 | 0.758 | 0.976 | 0.984 | 0.984 |
| 0.8 | 0.703 | 0.703 | 0.703 | 0.964 | 0.954 | 0.953 |
| 0.9 | 0.630 | 0.630 | 0.630 | 0.839 | 0.857 | 0.858 |
| 1.0 | 0.542 | 0.537 | 0.536 | 0.654 | 0.560 | 0.552 |
An example illustrating the effect of scattering on the solution obtained is shown in Fig. 1 for the square channel at Nappr = 0. At small optical thickness, the scattering changes only the distribution of emitted radiation, but at (1 - ω)τ0 = 1 it substantially reduces total radiation.

Figure 1. Effect of scattering on the medium emissivity in a square channel: 1 - (1 - ω)τ0 = 0.2, 2 - 0.4, 3 - 1.0; red lines - nonscattering medium, blue lines - ω = 0.5.
To estimate the maximum error of the diffusion approximation taking place in the corner regions, one can compare the analytical solution presented above with formulas derived by Zaltsman et al. (1985) for optically thin nonscattering medium (τ0 << 1) in a rectangular channel. According to Zaltsman et al. (1985), variation of the medium emissivity from the value at symmetry plane τ = 0 to that at the channel corner τ = τ0 is described by the function

|
(7a) |

|
(7b) |

|
(7c) |
A comparison of computational results obtained in the diffusion approximation and calculated by use of Eq. (7) is shown in Fig. 2.

Figure 2. Emissivity of a nonscattering medium in square channel: 1 - exact solution for optically thin medium; 2-5 - calculations in P1 approximation (2 - τ0 = 0.1, 3 - 0.2, 4 - 0.5, 5 -1).
The diffusion approximation gives a physically wrong result ε (τ) ≡ 1 within the limit of τ0 → 0. This is caused by an error of the Marshak boundary condition that supposes the radiation flux vector to be directed toward the normal to the boundary surface, only in this case integration of Eq. (5) provides Eq. (7). A turning of the radiation flux vector near the corner of the computational region is not taken into account by such a boundary condition. Therefore, the value of ε predicted by the diffusion approximation appears to be a factor of √2 greater than the exact value. It is interesting that the curves ε(τ) at τ0 = 0.5 - 1 are very close to the exact solution for optically thin medium. This result can be treated as a good qualitative description of the radiative transfer in a void by the diffusion approximation with the radiation diffusion coefficient D ~ L/ 2, where L is the characteristic size of the void, corresponding to the physical sense of the radiation diffusion coefficient. A similar estimate was used by Dombrovsky et al. (2007) in a diffusion-based model of a solar chemical reactor. The diffusion approximation gives functions ε(τ) having considerable reduction in the corner regions. The noted boundary condition error takes place, but more important is the decrease of radiation energy density toward a corner, which is satisfactory when calculated in the diffusion approximation. Conditions for diffusion approximation applicability are considerably improved by radiation scattering in the medium and diffuse wall reflection, as well as by thermal radiation of the walls, so that the error for the problem as a whole is expected to be much smaller.
Of course, the error of diffusion approximation decreases with the optical thickness, but calculations for an optically thick medium are more complicated and one needs additional simplifications of the computational model. Obviously, the correct value of ε (1) within the limit of an optically thick medium is 0.5. This limiting value can also be obtained in the diffusion approximation, but the solution convergence is very slow in the corner region (see Table 1).
REFERENCES
Abramowitz, M. and Stegun, I. A., (Eds.) Handbook of Mathematical Functions, New York: Dover Publications, 1965.
Dombrovsky, L. A. and Barkova, L. G., Solving the two-dimensional problem of thermal-radiation transfer in an anisotropically scattering medium using the finite element method, High Temp., vol. 24, no. 4, pp. 585-592, 1986.
Dombrovsky, L. A., Lipiński, W., and Steinfeld, A., A diffusion-based approximate model for radiation heat transfer in a solar thermochemical reactor, J. Quant. Spectrosc. Radiat. Transf., vol. 103, no. 3, pp. 601-610, 2007.
Zaltsman, I. G., Ishmaev, A. L., and Shikov, V. K., Radiative flux distribution over rectangular channel perimeters of power plants, High Temp., vol. 23, no. 4, pp. 766-770 (in Russian), 1985.
参考文献
- Abramowitz, M. and Stegun, I. A., (Eds.) Handbook of Mathematical Functions, New York: Dover Publications, 1965.
- Dombrovsky, L. A. and Barkova, L. G., Solving the two-dimensional problem of thermal-radiation transfer in an anisotropically scattering medium using the finite element method, High Temp., vol. 24, no. 4, pp. 585-592, 1986.
- Dombrovsky, L. A., Lipiński, W., and Steinfeld, A., A diffusion-based approximate model for radiation heat transfer in a solar thermochemical reactor, J. Quant. Spectrosc. Radiat. Transf., vol. 103, no. 3, pp. 601-610, 2007.
- Zaltsman, I. G., Ishmaev, A. L., and Shikov, V. K., Radiative flux distribution over rectangular channel perimeters of power plants, High Temp., vol. 23, no. 4, pp. 766-770 (in Russian), 1985.