A differential equation (DE) is a relationship between a function required (dependent variable), its derivatives of different orders, and independent variables. A DE describing a macroscopic physical process in continuum medium can be derived in two ways. The first is called phenomenological and based on mathematical representation of the corresponding physical law (or laws) for an elementary volume in a vicinity of a point in the space of independent variables, the representation being normalized by the value of the volume, followed by a formal transformation to the point limit. The other, statistical way involves a probabilistic approach to phenomena concerned with individual medium particles and deals with characteristics averaged over an ensemble of particles or process realizations.
Differential equations can be classified into ordinary differential equations containing derivatives with respect to one independent variable, and partial differential equations containing partial derivatives of the required function with respect to several independent variables. The order of the DE is the order of the highest derivative entering it. Every function substituted into a DE and reducing it to an identity is called its solution. The relationship L(φ) = f(t, x, y, z) is called a linear DE when the sum of two partial solutions, φ1 and φ2, of the homogeneous DE L(φ) = 0 is its solution too, i.e., L(φ1 +φ2) = 0, where L(j) is the linear operator of differentiation.
To complete the mathematical description of a nonsteady physical process, represented by a given DE, the initial values of the required function must be specified in the whole domain of its description at the initial time (for a partial DE). Also, the values of the function or (and) its derivatives of the order less than n on the domain boundary (or on its part), or alternatively relationships describing the physical processes of interest on the boundary, must be specified for the whole time interval. In the case of steady physical process, only boundary values need to be specified. The initial and boundary values form the boundary conditions, and the set of DEs and corresponding boundary conditions, the boundary problem.
The order of a set of DEs (SDE) is the sum of orders of all DEs entering the SDE. Generally, the number of equations and additional relationships between them have to be equal to a number of unknown functions.
The Cauchy problem for a second order partial DE consists of finding a solution in some neighborhood of the smooth surface Г, where the values of the function and its derivative along some nontangent direction
 are specified.
are specified.

Functions φ0 and φ1 are the Cauchy data, and surface Г is the Cauchy surface.
Normally, the boundary conditions have to be specified so that the boundary problem would have a unique solution. Sometimes, it is necessary to prove the theorems of existence and uniqueness for a given problem. For the general description of the boundary problem in the form of equation Dφ = Ф, where D is the operator of the given boundary problem and Ф is the set of right-hand sides of the DE and boundary conditions, the uniqueness theorem is equivalent to the existence of reverse transformation D−1 and the existence theorem, which refer to the coincidence of the operator D results domain with the results domain of right hand side Ф. The problems of solution existence and uniqueness are especially important for nonlinear DE's.
For boundary problems, the important question is accuracy, besides the existence and uniqueness. Small variations on boundary conditions or DE coefficients, which can always take place due to some inaccuracy of physical quantity measurements, may lead to variations in boundary problem solution too.
Partial DEs of the second order form
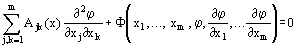
are classified according to the real characteristic values λi of the matrix (A) of the highest coefficients, which can be supposed to be symmetrical (it can always be achieved) and are obtained from the equation
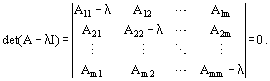
The equation is of the type (α, β, γ) in the given point x, if among characteristic values λi there are α positive, β negative and γ zero values (α + β + γ = m). Three types of such partial DE's take particular roles in the theory of fluid flow and heat and mass transfer. The type (m, 0, 0) = (0, m, 0) is called elliptic (for instance, the Laplace and Helmholtz equations); the type (m−1, 0, 1) = (0, m−1, 1), is parabolic (for instance, the diffusion equation); and the type (m−1, 1, 0) = (1, m−1, 0) is hyperbolic (for instance, the wave equation). Due to the fact that the majority of physical problems leads to such equations, the theory for this type of equation is more developed than the theory for other partial DE types.
The characteristic surfaces (characteristics) of a partial DE of the second order are defined by nontrivial solutions of the first order equation

The characteristics are invariant with respect to independent variable transformations. Elliptic equations do not have real characteristics. The parabolic type DE characteristics are planes xm = const (xm is a coordinate corresponding to γ = 1). The Cauchy data on characteristics are linked by some relationship. If it is violated, the Cauchy problem for a hyperbolic DE with boundary conditions on a characteristic has no solution. The boundary conditions for elliptic DE's are distinguished from those for hyperbolic DE's. For elliptic DE's, the problem is to find a function in the whole definition domain under the given function (or its normal derivative) value along the whole boundary (Dirichlet, Neumann or mixed problems). For hyperbolic DE, the problem is to find a function in the whole domain where its value, and the value of its derivative along some direction - nontangential to the boundary surface - is defined on part of, not the whole, boundary (Cauchy problem). For a DE of parabolic type, boundary conditions for the parabolic direction (Amn = 0) are defined on the characteristic xm = const. Depending on conditions, the same general physical process can be described by DEs of different types. For instance, a heat conduction process in solid body with constant physical properties and with sharp variation of conditions at its surface can be, at very short times (of the order of nanoseconds), of a wave character. It can be described by a hyperbolic DE, of the rectangular coordinate system, in the form

where τ is the heat relaxation time and κ is the thermal diffusivity. For moderate conditions, when a vector of heat flux density can be described by the Fourier law
 = −λ gradT, the equation is of the parabolic type and takes the form
= −λ gradT, the equation is of the parabolic type and takes the form

Under steady-state boundary conditions, the steady temperature distribution (if it exists) is described by the elliptic Laplace equation

Cases where analytical solutions exist for partial DE and SDE are very rare, and related mainly to linear problems and to conditions when a solution has a self-similar character—i.e., when an independent variable can be expressed as a complex of initial independent variables, leading to a reduction of the independent variables' number.
Among the analytical methods of partial DE solution, the following are the most widely employed: separation of variables method, where the solution is (if it is possible) in the form of a product of two or more functions of different independent variables (thus the problem is reduced to solving two or more DE's, depending on the reduced set of independent variables); methods of integral transforms, including Laplace, Fourier and other transforms; method of Green's functions; method of asymptotic expansions (mainly matched asymptotic expansions); variational method (for instance, the Bubnov-Galerkin method), where the solution of a problem—described by a partial DE and boundary and initial conditions—is obtained by solving a minimization problem for the corresponding functional.
In recent years, numerical methods have been widelyemployed for the solution of problems described by partial DE and SDE; these include finite-differences, finite elements and boundary elements.