RADIATIVE EFFECTS IN A SEMI-TRANSPARENT LIQUID CONTAINING GAS BUBBLES
Following from: Radiative transfer problems in nature and engineering
The problem treated in this section arises in the case of water cooling of very hot surfaces. A practical example is provided by the delivery of water to the surface of the corium pool in the case of a nuclear reactor severe accident as reported by Bechta et al. (2000). Because of high temperature (2000-3000 K), the main part of the thermal radiation of the pool surface is in the near infrared, where water is semi-transparent. As a result, a considerable part of the radiation is absorbed not in the thin surface layer of water but in the volume far enough from the interface (Dombrovsky, 2003, 2004). The volume heat source can lead to generation of vapor bubbles, which affect the radiation transfer. In the complete physical problem statement, one should take into account this feedback relation and time evolution of a two-phase medium structure. In the present analysis, we focus on the radiative part of the general problem: the effect of bubbles on the radiative properties of water and radiative transfer in this two-phase system taking into account radiation scattering. The radiation problem to be solved is interesting not only for liquids but also for semi-transparent solids with numerous gas bubbles or other spherical particles. Similar structures arise in glass production and are used in some thermal insulation materials (Richter et al., 1995; Bynum, 2001; Fedorov and Pilon, 2002; Shelby, 2005). The correct radiation transfer models for such media are very important for industrial applications (Baillis and Sacadura, 2000).
The following assumptions are used to determine the radiative properties of a medium with bubbles: the radiation is absorbed in a layer, in which the thickness is much greater than the bubble sizes; the bubbles are spherical; the bubbles are randomly positioned in the volume; and the distances between the bubbles are much greater that their sizes and radiation wavelengths.
The first assumption denotes that we consider only a range of semi-transparency. Assumptions concerning random location and a concentration of bubbles that is not too high allow ignoring effects of dependent scattering (Tien and Drolen, 1987). The restriction of our treatment to bubbles of spherical shape simplifies significantly the determination of their radiative characteristics. The properties of single gas bubbles in a semi-transparent liquid or solid medium and the effect of monodisperse bubbles on radiation absorption and scattering by the medium were considered in the article Radiative properties of gas bubbles in semi-transparent medium. In the case of large bubbles with a radius of a >> λ, the following simple relations for absorption and transport scattering coefficients appeared to be true:

|
(1) |
where fv is the volume fraction of bubbles and αλ,e = 4πκe/ λ is the absorption coefficient of the host medium characterized by optical constants ne and κe. In the case of polydisperse bubbles, only the equation for σλtr is slightly modified:

|
(2) |
where F(a) is the size distribution of bubbles and a32 is the Sauter mean radius of the bubbles. One can see that the role of scattering is determined by the ratio of the bubble volume fraction and their average radius, fv/ a32.
Radiative Transfer Model
Consider the one-dimensional radiative transfer problem in a semi-infinite layer of an absorbing, refracting, and scattering medium, illuminated uniformly by diffuse randomly polarized external radiation. In transport approximation, the radiative transfer equation (RTE) and boundary condition can be written as follows:

|
(3a) |

|
(3b) |
Here, μ = cosθ, θ is measured from the normal directed to the medium, qλe is the spectral external radiation flux, and R(μ) is Fresnel’s reflection coefficient. It is assumed that surface z = 0 is optically smooth. In the case of nonscattering medium, one can obtain the following analytical solution to the problem (3):

|
(4) |
where Θ is the Heaviside unit step function. According to Eq. (4), the radiation--after entering a refractive medium--propagates within a cone with an apex angle of 2cos-1(μc). Equation (4) was used by Dombrovsky (2003) for a liquid without bubbles. The radiation power absorbed in the medium, P(z), was defined as:

|
(5) |
where the spectral radiation energy density is

|
(6) |
In the presence of scattering, the radiation field in the medium becomes significantly more complex. If scattering prevails over absorption, the angular dependence of the radiation intensity does not exhibit the feature mentioned above and the characteristic of the solution in a nonscattering medium. In other words, there is no sharp angular variation of the radiation intensity near the cone angle in a strongly scattering medium, which means that one of the known differential approximations can be used as was done by Fedorov and Viskanta (2000). However, in the general case of an arbitrary ratio between absorption and scattering, this may lead to a significant underestimation of the depth of radiation penetration. Therefore, in the paper (Dombrovsky, 2004) the author followed (Dombrovsky, 1996a,b) and suggested a combined solution in which the differential approximation is used only to determine the integral term on the right-hand side of the RTE [Eq. (3a)] and, at the second step of the solution, the RTE with the known right-hand side is integrated.
As a differential approximation of the first step, we use DP0 (the two-flux approximation). The corresponding boundary-value problem is written as

|
(7a) |

|
(7b) |

|
(7c) |
Here, Dλ = 1/(4 βλtr) is the spectral radiation diffusion coefficient. Following Siegel and Howell (2002), the coefficient in the boundary condition at the interface is determined using the normal reflection coefficient R(1) = (n - 1)2/ (n + 1)2. The solution to problem (7) gives the approximate profile of the spectral radiation energy density for the right-hand side of Eq. (3a). Note that problem (7) has a simple analytical solution at constant coefficients αλ and Dλ:

|
(8) |
One can estimate an error of DP0 in the case of weakly scattering medium. It is sufficient to compare Eqs. (6) and (8) at βλtr = αλ.
The solution to Eq. (3a) with the right-hand side determined in the DP0 approximation can be obtained using an integrating factor:
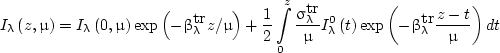
|
(9a) |

|
(9b) |
Equations (9a) and (9b) in combination with boundary condition (3b) make it possible to calculate the spectral radiation intensity in all directions at any point of the computational region. First, Iλ(z,-μ) is determined by Eq. (9b), and then the known value of Iλ(0,-μ) is used to calculate Iλ(0,μ) from boundary condition (3b). After that, Iλ(z,μ) is calculated by Eq. (9a).
However, it is not our objective to determine the angular dependence of the spectral radiation intensity. It is sufficient to calculate the new (second-step) approximate profile of the spectral radiation energy density:

|
(10) |
where

|
(11a) |

|
(11b) |

|
(11c) |
Equations (10) and (11) enable us to calculate a more accurate value of the spectral radiation energy density. The integro-exponential functions appearing in Eq. (11) are defined as

|
(12a) |

|
(12b) |
Formula (12a) corresponds to the regular definition (Özişik, 1973; Siegel and Howell, 2002), and Eq. (12b) is distinguished by a nonzero lower integration limit.
After determining Iλ0,new(z), the radiation power absorbed in the medium, P(z), is calculated by integration over the spectrum according to Eq. (5). Note that the function P(z) satisfies the following energy balance equation:

|
(13) |
where q is the integral radiation flux on the interface z = 0. We will follow the papers by Dombrovsky (2003, 2004) and, along with the differential characteristic of absorption P(z), use the function corresponding to the fraction of total integral flux of thermal radiation absorbed in a layer (0,z):

|
(14) |
Some Results for Water with Steam Bubbles
Before proceeding to a model radiative transfer problem, one should remember that our approach is applicable only in the spectral range of water semi-transparency. In this range, water is a weakly absorbing substance and the ordinary radiative transfer model can be employed. In the long-wave spectral range of water opacity, the problem degenerates and the respective part of the external radiation is totally absorbed in a thin surface layer of water.
Consider a model problem of radiative transfer in a thick layer of water containing steam bubbles. The spectrum of radiation incident on the water layer is taken to be similar to the spectrum of blackbody radiation for some temperature Te; i.e., it is assumed that qλe = ελBλ(Te) and ελ = const. The volume fraction of steam bubbles and their size are assumed constant over the entire layer of water. In this case, coefficients αλ, σλtr, and βλtr are independent of coordinate z.
It goes without saying that the real problem is conjugate, because the profiles of bubble volume fraction fv(z) and average bubble radius a32(z) affect the radiation transfer, and the volumetric absorption of radiation causes a variation of the fv(z) and a32(z) profiles. For closing the problem, one should use some kinetic model describing the nucleation and growth of steam bubbles with due regard to the absorption of thermal radiation. Such a general problem is beyond the scope of this study; therefore, in the model calculations performed the constant values of fv and a32 are treated as preassigned parameters.
In the calculations of the spectral radiative properties of water containing steam bubbles, the infrared index of absorption of water is determined by interpolation of tabular data by Hale and Querry (1973). The index of refraction is taken to be ne = 1.33 (in the range of water semi-transparency it varies from 1.335 at λ = 0.5 μm to 1.324 at λ = 1.2 μm). The steam in the bubbles is assumed to be transparent for thermal radiation. The results of the calculations of dependencies Q(z) for different constant values of ratio fv/ a32 are shown in Fig. 1. Comparison of the results obtained using the combined model suggested and the DP0 calculations without the second step of solution shows that DP0 gives qualitatively correct results but underestimates significantly the thickness of the absorbing water layer. The error of the DP0 approximation somewhat decreases with increasing the contribution of scattering. As it was expected, the scattering of radiation by steam bubbles leads to the absorption of radiation in a thinner layer of water. This effect becomes significant even at fv/a32 ≥ 10m-1.

Figure 1. Effect of the steam bubbles on the absorption of radiation in the water layer: (a) - Te = 2000 K, (b) - Te = 3000 K; I - DP0 approximation, II - combined two-step model; 1 - fv/a32 = 0, 2 - fv/a32 = 10 m-1, 1 - fv/a32 = 100 m-1, 4 - fv/a32 = 1000 m-1.
Figure 2 illustrates the effect of relatively low values of the parameter fv/a32 on thickness Δ of the water layer in which the bulk of the power of thermal radiation is absorbed at Te = 3000 K. The plotted values of Δ were determined from Q(Δ) = 0.7 and Q(Δ) = 0.8. In order to understand how real the pre-assigned values of fv/a32 are, one can turn to the experimental data by Hibiki and Ishii (2002) and Hibiki et al. (2003), according to which the value of fv/a32 in the majority of cases varies from 5 to 100. In this range, the treated effect shows up quite clearly.

Figure 2. Effect of the steam bubbles on the thickness of the water layer in which (1) 70% and (2) 80% of the radiation power is absorbed: calculation using the combined model, Te = 3000 K.
Turning back to the discussion of the conjugate problem in view of the effect of the radiation absorption on the nucleation and growth of steam bubbles (bearing in mind, for example, film boiling on a surface with a temperature above 2000 K), note the presence of positive feedback: an increase in the volume fraction and the size of the bubbles leads to an ever stronger absorption of radiation in a thin layer of water in the vicinity of the surface. At the same time, the total radiation power absorbed in the water layer decreases because of the reflection of radiation from water containing vapor bubbles. As a result, the role of the bubbles in the interaction of thermal radiation with water is not so simple and it should be considered on the basis of more realistic model problems.
Consider the model problem of volume heating of a thick water layer by external thermal radiation. The initial temperature of water is assumed constant and equal to the saturation temperature at atmospheric pressure. The temperature profile T(t,z) in the water layer can be calculated by solving the following transient heat conduction problem:

|
(15a) |

|
(15b) |

|
(15c) |
where zi is the coordinate of the moving steam/water interface. The profile of absorbed radiation power P(t,z) depends on the current profile of bubble concentration parameter fv/ a32. Possible convective heat transfer in the water layer is not taken into account.
One can assume that vapor bubbles appear only at great overheating of water. The results of the numerical calculations for pure water without steam bubbles are presented in Fig. 3, where ΔT = T - Tsat.

Figure 3. Temperature profiles in water by radiative heating: (a) - Te = 2500 K, (b) - Te = 3000 K.
Note that maximum overheating (ΔT)max can be found from the homogeneous nucleation theory (Skripov, 1974). For water at atmospheric pressure we have the value of (ΔT)max = 320 K. The calculations showed that maximum overheating is reached at t = 13.8 s when Te = 2500 K and at t = 3.6 s when Te = 3000 K. Intensive generation of vapor bubbles is expected in the region near the maximum of the temperature. The modeling of the generation and growth of bubbles is beyond the scope of our study. But it is interesting to consider the radiation transfer for realistic profiles of bubble concentration. For this reason, the following relation between parameter fv/a32 and local overheating ΔT is assumed:

|
(16) |
In the numerical procedure, the radiative transfer has been calculated at each time step of solving the transient conduction problem [Eq. (15)]. Some results of the calculations are shown in Fig. 4.

Figure 4. Temperature profiles in the water layer by radiative heating at Te = 3000 K: I - without bubbles (fv = 0), II - (fv/a32)max = 104 m-1; 1 - t = 0.5 s, 2 - t = 1 s, 3 - t = 2 s.
The radiation scattering by steam bubbles does not practically affect the evaporation rate and the position of the temperature maximum. At the same time, the maximum overheating of water decreases considerably. Remember that overheating decreases also due to evaporation heat. Nevertheless, significant overheating may result in explosive volume evaporation of water with generation of sprays of fine water droplets toward the illuminated steam/water interface. This process is expected to be periodical and its effect on the net heat transfer rate may be significant. One can imagine two stages of the process: (1) water heating and evaporation before explosion-like behavior with generation of a layer of droplets; and (2) heating and evaporation of droplets, which shield the water layer from thermal radiation. In the case of Te = 3000 K, the predicted duration of the first stage of the process for pure water is about 2-3 s and the mass of dispersed water per unit surface of the interface can be estimated as ρδ, where δ ≈ 2 mm is the distance between the interface and the region of maximum overheating. To develop a complete theoretical model one should also consider the radiation shielding by evaporated water droplets. It goes without saying, the latter physical model of explosive evaporation should be verified experimentally.
REFERENCES
Baillis, D. and Sacadura, J.-F., Thermal radiation properties of dispersed media: theoretical prediction and experimental characterization, J. Quant. Spectrosc. Radiat. Transf., vol. 67, no. 5, pp. 327-363, 2000.
Bechta, S. V., Vitol, S. A., Krushinov, E. V., Granovsky, V. S., Sulatsky, A. A., Khabensky, V. B., Lopukh, D. B., Petrov, Yu. B., and Pechenkov, A. Yu., Water boiling on the corium melt surface under VVER severe accident conditions, Nucl. Eng. Des., vol. 195, no. 1, pp. 45-56, 2000.
Bynum, R. T., Jr., Insulation Handbook, New York: McGraw-Hill, 2001.
Dombrovsky, L. A., Radiation Heat Transfer in Disperse Systems, New York: Begell House, 1996a.
Dombrovsky, L. A., Approximate methods for calculating radiation heat transfer in dispersed systems, Therm. Eng., vol. 43, no. 3, pp. 235-243, 1996b.
Dombrovsky, L. A., Radiation transfer through a vapour gap under conditions of film boiling of liquid, High Temp., vol. 41, no. 6, pp. 819-824, 2003.
Dombrovsky, L. A., The propagation of infrared radiation in a semitransparent liquid containing gas bubbles, High Temp., vol. 42, no. 1, pp. 133-139, 2004.
Hale, G. M. and Querry, M. P., Optical constants of water in the 200 nm to 200 μm wavelength region, Appl. Opt., vol. 12, no. 3, pp. 555-563, 1973.
Hibiki, T. and Ishii, M., Development of one-group interfacial area transport equation in bubbly flow systems, Int. J. Heat Mass Transfer, vol. 45, no. 11, pp. 2351-2372, 2002.
Hibiki, T., Situ, R., Mi, Y., and Ishii, M., Modeling of bubble-layer thickness for formulation of one-dimensional interfacial area transport equation in subcooled boiling two-phase flow, Int. J. Heat Mass Transfer, vol. 46, no. 8, pp. 1409-1423, 2003.
Fedorov, A. G. and Viskanta, R., Radiative transfer in a semitransparent glass foam blanket, Phys. Chem. Glasses, vol. 41, no. 3, pp. 127-135, 2000.
Fedorov, A. G. and Pilon, L., Glass foams: Formation, transport properties, and heat, mass, and radiation transfer, J. Non-Cyst. Solids, vol. 311, no. 2, pp. 154-173, 2002.
Özişik, M. N., Radiative Transfer and Interaction with Conduction and Convection, New York: Wiley, 1973.
Richter, K., Norris, P. M., and Tien, C.-L., Aerogels: Applications, structure, and heat transfer phenomena, Annu. Rev. Heat Transfer, vol. 6, pp. 61-114, 1995.
Shelby, J. E., Introduction to Glass Science and Technology, 2nd ed., Cambridge, UK: The Royal Society of Chemistry, 2005.
Siegel, R. and Howell, J. R., Thermal Radiation Heat Transfer, 4th ed., New York: Taylor & Francis, 2002.
Skripov, V. P., Metastable Liquids, New York: Wiley, 1974.
Tien, C. L. and Drolen, B. L., Thermal radiation in particulate media with dependent and independent scattering, in Annual Review of Numerical Fluid Mechanics and Heat Transfer, vol. 1, pp. 1-32, New York: Hemisphere, 1987.
References
- Baillis, D. and Sacadura, J.-F., Thermal radiation properties of dispersed media: theoretical prediction and experimental characterization, J. Quant. Spectrosc. Radiat. Transf., vol. 67, no. 5, pp. 327-363, 2000.
- Bechta, S. V., Vitol, S. A., Krushinov, E. V., Granovsky, V. S., Sulatsky, A. A., Khabensky, V. B., Lopukh, D. B., Petrov, Yu. B., and Pechenkov, A. Yu., Water boiling on the corium melt surface under VVER severe accident conditions, Nucl. Eng. Des., vol. 195, no. 1, pp. 45-56, 2000.
- Bynum, R. T., Jr., Insulation Handbook, New York: McGraw-Hill, 2001.
- Dombrovsky, L. A., Radiation Heat Transfer in Disperse Systems, New York: Begell House, 1996a.
- Dombrovsky, L. A., Approximate methods for calculating radiation heat transfer in dispersed systems, Therm. Eng., vol. 43, no. 3, pp. 235-243, 1996b.
- Dombrovsky, L. A., Radiation transfer through a vapour gap under conditions of film boiling of liquid, High Temp., vol. 41, no. 6, pp. 819-824, 2003.
- Dombrovsky, L. A., The propagation of infrared radiation in a semitransparent liquid containing gas bubbles, High Temp., vol. 42, no. 1, pp. 133-139, 2004.
- Hale, G. M. and Querry, M. P., Optical constants of water in the 200 nm to 200 μm wavelength region, Appl. Opt., vol. 12, no. 3, pp. 555-563, 1973.
- Hibiki, T. and Ishii, M., Development of one-group interfacial area transport equation in bubbly flow systems, Int. J. Heat Mass Transfer, vol. 45, no. 11, pp. 2351-2372, 2002.
- Hibiki, T., Situ, R., Mi, Y., and Ishii, M., Modeling of bubble-layer thickness for formulation of one-dimensional interfacial area transport equation in subcooled boiling two-phase flow, Int. J. Heat Mass Transfer, vol. 46, no. 8, pp. 1409-1423, 2003.
- Fedorov, A. G. and Viskanta, R., Radiative transfer in a semitransparent glass foam blanket, Phys. Chem. Glasses, vol. 41, no. 3, pp. 127-135, 2000.
- Fedorov, A. G. and Pilon, L., Glass foams: Formation, transport properties, and heat, mass, and radiation transfer, J. Non-Cyst. Solids, vol. 311, no. 2, pp. 154-173, 2002.
- ÖziÅŸik, M. N., Radiative Transfer and Interaction with Conduction and Convection, New York: Wiley, 1973.
- Richter, K., Norris, P. M., and Tien, C.-L., Aerogels: Applications, structure, and heat transfer phenomena, Annu. Rev. Heat Transfer, vol. 6, pp. 61-114, 1995.
- Shelby, J. E., Introduction to Glass Science and Technology, 2nd ed., Cambridge, UK: The Royal Society of Chemistry, 2005.
- Siegel, R. and Howell, J. R., Thermal Radiation Heat Transfer, 4th ed., New York: Taylor & Francis, 2002.
- Skripov, V. P., Metastable Liquids, New York: Wiley, 1974.
- Tien, C. L. and Drolen, B. L., Thermal radiation in particulate media with dependent and independent scattering, in Annual Review of Numerical Fluid Mechanics and Heat Transfer, vol. 1, pp. 1-32, New York: Hemisphere, 1987.