Hemispherical Transmittance and Reflectance at Normal Incidence
Following from: The simplest approximations of double spherical harmonics, Solutions for one-dimensional radiative transfer problems
The measurements of directional-hemispherical transmittance and reflectance are widely used in present-day identification procedures for obtaining information on the main radiative properties of semi-transparent disperse media (Baillis and Sacadura, 2000; Sacadura and Baillis, 2002). According to the radiation transfer theory, the transmittance and reflectance of a homogeneous sample of thickness d depend on the following spectral parameters of the medium: index of refraction nλ, optical thickness τλ0 = βλd, albedo ωλ, and scattering function Φλ. Here, we will omit subscript λ in designations of spectral values. It is clear that it is difficult to determine all values n, ω, τ0, and Φ on the basis of directional-hemispherical measurements. Fortunately, the index of refraction is usually known from independent measurements and the effect of the scattering function on hemispherical transmittance and reflectance can be described by the transport approximation. The applicability of the transport approximation for problems with collimated incident radiation is not evident. It has been recently analyzed by Dombrovsky et al. (2005, 2006, 2007). A modification of the two-flux method applicable for the case of collimated irradiation of a refracting sample was also proposed in these studies. After separation of the collimated and diffuse components of the radiation field, the angular dependence of the diffuse radiation intensity is approximated in this method by a step function referenced to the angle of total internal reflection at the interface.
Consider the problem of radiation transfer in a plane-parallel layer of an absorbing, refracting, and scattering medium. We will limit our consideration to the one-dimensional azimuthally symmetric problem in which one surface of the layer is uniformly illuminated along the normal direction by randomly polarized radiation. In the case of homogeneous isotropic medium, the radiative transfer equation (RTE) and the associated boundary conditions can be written as follows:

|
(1) |
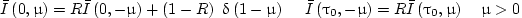
|
(1b) |
where I = Iλ/(n2,Ie), Ie is the incident spectral radiation intensity, and R(μ) is the Fresnel’s reflection coefficient (Born and Wolf, 1999; Modest, 2003):

|
(2a) |

|
(2b) |
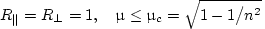
|
(2c) |
We assume here that the index of absorption is relatively small: κ << n. The value of R = 1 for μ ≤ μc corresponds with the total internal reflection.
Note that similar radiative transfer problems for a plane-parallel layer of a refracting medium have been considered by Armaly and Lam (1975), Spiga et al. (1980), and Santarelli et al. (1980). It was shown that hemispherical reflectance and transmittance can be determined on the basis of simplified differential models such as exponential kernel approximation. The latter model is equivalent to the ordinary diffusion approximation (Özişik, 1973; Modest, 2003). One should also remember the results obtained by Modest (1991) for oblique collimated irradiation of a plane-parallel layer of a nonrefracting medium. It was proven by Modest (1991) that the P1 approximation gives good results for the scattered radiation field.
Let us return to problem (1). In the transport approximation, this problem is reduced to the following:

|
(3a) |
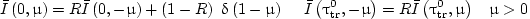
|
(3b) |
Following the usual technique (Sobolev, 1972, 1975; Dombrovsky, 1996; Modest, 2003) with the present radiation intensity I as a sum of the diffuse component J and the term that corresponds to the transmitted and reflected directional external radiation:

|
(4) |
where Ctr = R1 exp(-2τtrO) and R1 = R(1). The index of absorption of the host medium is assumed to be small in comparison to the index of refraction. In this case, one can use approximate formula R1 = (n - 1)2/(n + 1)2.
The mathematical problem statement for the diffuse component of radiation intensity is as follows:

|
(5a) |

|
(5b) |
The directional-hemispherical reflectance and transmittance can also be expressed through the diffuse component of the radiation intensity:

|
(6) |
where the first terms are given by the well-known equations (Modest, 2003):

|
(7) |
It is important that the source term on the right-hand side of Eq. (5a) does not depend on angular coordinate μ. This enables the further simplification of the problem. Note that separation of collimated radiation in the case of arbitrary scattering function [problem (1)] leads to the following relations instead of Eqs. (3) and (5a):

|
(8a) |

|
(8b) |
where

|
(9) |
The source function on the right-hand side of Eq. (8b) depends on μ and it makes the problem much more complicated than that for the transport approximation.
In the case of spherical particles or long fibers with a known size distribution, one can use the Mie theory to predict the scattering function of the medium (van de Hulst, 1957, 1981; Bohren and Huffman, 1983). In many other cases, the scattering functions of a disperse medium are unknown and it is difficult to find the transport approximation error. We will assume that this error can be evaluated by comparison of the calculations for two model scattering functions: the transport one and the Henyey-Greenstein function (see the article Transport Approximation). It is clear that the difference between these two approximations is negligible at a small asymmetry factor and increases with μ mainly because of quite different backward scattering. The latter is expected to be important for calculating the reflectance. Note that some real scattering functions cannot be well approximated by the Henyey-Greenstein function and one needs a more adequate approximation for exact radiation transfer calculations. In these cases, as has been shown by Tagne and Baillis (2005), the transport approximation may be even better than the Henyey-Greenstein one.
Numerical solution for the RTE at both transport and Henyey-Greenstein scattering functions can be obtained by use of the discrete ordinates method (DOM). It is known that Fresnel’s reflection may cause the so-called ray effect associated with insufficiently fine angular discretization of the radiation intensity field (Coelho, 2002). Ray effects may be mitigated by refining the angular discretization or by using modifications of the DOM. In our case, an adequate account of the angular dependence can be reached by use of the composite DOM (CDOM) when the integral over the direction is split into integrals over three subintervals by the critical angle, and each subinterval uses a set of quadrature points (Liou and Wu, 1996; Wu and Liou, 1997). Note that a similar numerical method was used by Muresan et al. (2004) for the radiative-conductive heat transfer problem in nonscattering medium.
The effect of the scattering function on directional-hemispherical transmittance and reflectance is illustrated by the CDOM calculations presented in Figs. 1 and 2. The large value of μ is chosen to evaluate the upper limit of the difference between two approximations of the scattering function. One can see that the results depend considerably on the index of refraction. For nonrefracting medium (n = 1), the effect of the scattering function on transmittance is very small in comparison with the effect on reflectance. In the case of n = 1.4, the value of Td-h is more sensitive to the scattering function, whereas the results for Rd-h obtained in the transport approximation and in the Henyey-Greenstein approximation are closer to each other.

Figure 1. Effect of the scattering function on directional-hemispherical transmittance and reflectance. The CDOM calculations for nonrefracting medium: (a) transport approximation and (b) Henyey-Greenstein approximation for μ = 0.8; (1) τtr0 = 0.2, (2) τtr0 = 0.5, (3) τtr0 = 1.0, (4) τtr0 = 2.0, and (5) τtr0 = 5.0.

Figure 2. Effect of the scattering function on directional-hemispherical transmittance and reflectance. The CDOM calculations for n = 1.4: (a) transport approximation and (b) Henyey-Greenstein approximation for μ = 0.8; (1) τtr0 = 0.2, (2) τtr0 = 0.5, (3) τtr0 = 1.0, (4) τtr0 = 2.0, and (5) τtr0 = 5.0.
Assuming the scattering function is equal to the Henyey-Greenstein (HG) one, consider the value of relative errors of the transport approximations εT = Td-htr/Td-hHG - 1 and εR = Rd-htr/Rd-hHG - 1, where the superscripts denote the calculations for the corresponding scattering functions. The dependences of εT, εR on albedo and optical thickness shown in Fig. 3 confirm the results for the isotropic scaling accuracy obtained by Tagne and Baillis (2005) in the case of nonrefracting media. The data for sensitivity of the directional-hemispherical transmittance and reflectance to the scattering function should be taken into account in the procedure of identification of the radiative properties.
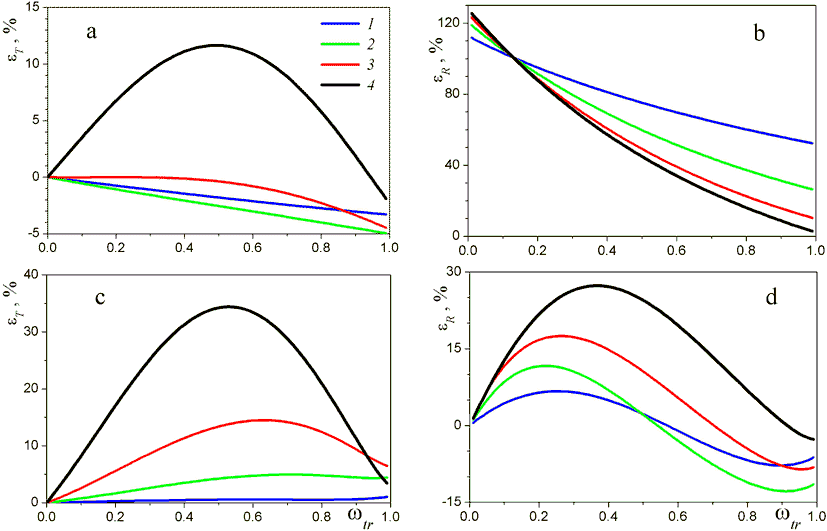
Figure 3. Relative error of the transport approximation for the case of Henyey-Greenstein scattering function μ = 0.8: (a) and (b) n = 1; (c) and (d) n = 1.4; (1) τtr0 = 0.2, (2) τtr0 = 0.5, (3) τtr0 = 1.0, and (4) τtr0 = 2.0.
The numerical procedure based on the CDOM code is general and can be applied to rather complicated problems. But, in the case of the transport scattering function, the angular dependencies of the diffuse radiation component are expected to be rather simple. For this reason, we consider an alternative approach, which is a modification of the well-known two-flux approximation. Taking into account the effect of total internal reflection on both interfaces of the medium layer, we suggest the following approximation (Dombrovsky et al., 2005, 2006):

|
(10) |
Note that in this case μc = 0 corresponds to the usual two-flux model. The intermediate angle interval -μc < μ < μc gives no contribution to the radiation flux and the words “two-flux” are applicable to the modified approximation as well. It is clear that relation (10) is just the same as in the CDOM of zero-order quadrature.
Integrating Eq. (5a) separately over the intervals -1 < μ < -μc, -μc < μ < μc, and μc < μ < 1, after simple transformations, one can obtain the following boundary-value problem for function g0 = φ0- + φ0+:

|
(11a) |

|
(11b) |
where

|
(12) |
Approximate equations for the reflectance and transmittance of the medium are written as:

|
(13) |
Boundary-value problem (11) can be solved analytically. Note that the particular solutions of inhomogeneous equation (11a) are as follows:
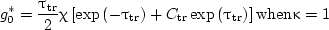
|
(14a) |

|
(14b) |
The resulting expressions for Rd-h and Td-h are different for κ = 1 and κ ≠ 1. In the first case, we have:
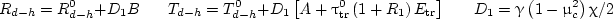
|
(15a) |

|
(15b) |

|
(15c) |
In the second case, Eq. (15b) is the same but Eqs. (15a) and (15c) should be replaced by the following:

|
(16a) |

|
(16b) |
In Eqs. (15a)-(15c), (16a), and (16b), the following designations are used:

|
(17) |
A comparison between the analytical solution (14)-(17) and the numerical results obtained by use of the high-order CDOM for the transport scattering function is given in Figs. 4 and 5. One can see that the modified two-flux approximation is rather accurate for both refracting and nonrefracting media, especially in the case of small and moderate optical thickness.

Figure 4. Directional-hemispherical transmittance and reflectance for the case of nonrefracting medium. Comparison of calculations by use of the modified two-flux approximation (red lines) with the exact numerical solution for transport scattering function (black lines): (1) τtr0 = 0.2, (2) τtr0 = 0.5, (3) τtr0 = 1.0, (4) τtr0 = 2.0, and (5) τtr0 = 5.0.

Figure 5. Directional-hemispherical transmittance and reflectance for the case of refracting medium n = 1.4. Comparison of calculations by use of the modified two-flux approximation (red lines) with the exact numerical solution for transport scattering function (black lines): (1) τtr0 = 0.2, (2) τtr0 = 0.5, (3) τtr0 = 1.0, (4) τtr0 = 2.0, and (5) τtr0 = 5.0.
A comparison of the corresponding analytical solution with the exact numerical calculations for the model transport scattering function showed that the error of the modified two-flux approximation is not greater than 5% in the most important range of the problem parameters. The wide-range calculations by use of the composite discrete ordinate method and the Henyey-Greenstein scattering function enables estimating the conditions when directional-hemispherical characteristics are insensitive to the scattering function and the transport approximation is applicable.
REFERENCES
Armaly, B. F. and Lam, T. T., Influence of refractive index on reflectance from a semi-infinite absorbing-scattering medium with collimated incident radiation, Int. J. Heat Mass Transfer, vol. 18, no. 7-8, pp. 893-900, 1975.
Baillis, D. and Sacadura, J.-F., Thermal radiation properties of dispersed media: theoretical prediction and experimental characterization, J. Quant. Spectrosc. Radiat. Transf., vol. 67, no. 5, pp. 327-363, 2000.
Bohren, C. F. and Huffman, D. R., Absorption and Scattering of Light by Small Particles, New York: Wiley, 1983.
Born, M. and Wolf, E., Principles of Optics, 7th ed., New York: Cambridge University Press, 1999.
Coelho, P. J., Bounded skew high order resolution schemes for the discrete ordinates method, J. Comput. Phys., vol. 175, no. 2, pp. 412-437, 2002.
Dombrovsky, L. A., Radiation Heat Transfer in Disperse Systems, New York: Begell House, 1996.
Dombrovsky, L., Randrianalisoa, J., Baillis, D., and Pilon, L. Use of Mie theory to analyze experimental data to identify infrared properties of fused quartz containing bubbles, Appl. Opt., vol. 44, no. 33, pp. 7021-7031, 2005.
Dombrovsky, L., Randrianalisoa, J., and Baillis, D., Modified two-flux approximation for identification of radiative properties of absorbing and scattering media from directional-hemispherical measurements, J. Opt. Soc. Am. A, vol. 23, no. 1, pp. 91-98, 2006.
Dombrovsky, L. A., Tagne, H. K., Baillis, D., and Gremillard, L., Near-infrared radiative properties of porous zirconia ceramics, Infrared Phys. Technol., vol. 51, no. 1, pp. 44-53, 2007.
Liou, B.-T. and Wu, C.-Y., Radiative transfer in a multi-layer medium with Fresnel interfaces, Heat Mass Transfer, vol. 32, no. 1-2, pp. 103-107, 1996.
Modest, M. F., Oblique collimated irradiation of an absorbing, scattering, plane-parallel layer, J. Quant. Spectrosc. Radiat. Transf., vol. 45, no. 5, pp. 309-312, 1991.
Modest, M. F., Radiative Heat Transfer, 2nd ed., New York: Academic, 2003.
Muresan, C., Vaillon, R., Menezo, C., and Morlot, R., Discrete ordinates solution of coupled conductive radiative heat transfer in a two-layer slab with Fresnel interfaces subject to diffuse and obliquely collimated irradiation, J. Quant. Spectrosc. Radiat. Transf., vol. 84, no. 4, pp. 551-562, 2004.
Özişik, M. N., Radiative Transfer and Interaction with Conduction and Convection, New York: Wiley, 1973.
Sacadura, J.-F. and Baillis, D., Experimental characterization of thermal radiation properties of disperse media, Int. J. Therm. Sci., vol. 41, no. 7, pp. 699-707, 2002.
Santarelli, F., Stramigioli, C., and Spiga, G., Rigorous and simplified approach to the radiative transfer in an absorbing and anisotropically scattering slab with a reflecting boundary, Int. J. Heat Mass Transfer, vol. 23, no. 6, pp. 853-860, 1980.
Sobolev, V. V., Light Scattering in Planetary Atmospheres, Moscow: Nauka (in Russian), 1972.
Sobolev, V. V., Light Scattering in Planetary Atmospheres, Oxford, UK: Pergamon, 1975.
Spiga, G., Santarelli, F., and Stramigioli, C., Radiative transfer in an absorbing and anisotropically scattering slab with a reflecting boundary, Int. J. Heat Mass Transfer, vol. 23, no. 6, pp. 841-852, 1980.
Tagne, H. T. K. and Baillis, D., Isotropic scaling limits for one-dimensional radiative heat transfer with collimated incidence, J. Quant. Spectrosc. Radiat. Transf., vol. 93, no. 1-3, pp. 103-113, 2005.
van de Hulst, H. C., Light Scattering by Small Particles, New York: Wiley, 1957.
van de Hulst, H. C., Light Scattering by Small Particles, New York: Dover, 1981.
Wu, C.-Y. and Liou, B.-T., Discrete-ordinate solutions for radiative transfer in a cylindrical enclosure with Fresnel boundaries, Int. J. Heat Mass Transfer, vol. 40, no. 10, pp. 2467-2475, 1997.
References
- Armaly, B. F. and Lam, T. T., Influence of refractive index on reflectance from a semi-infinite absorbing-scattering medium with collimated incident radiation, Int. J. Heat Mass Transfer, vol. 18, no. 7-8, pp. 893-900, 1975.
- Baillis, D. and Sacadura, J.-F., Thermal radiation properties of dispersed media: theoretical prediction and experimental characterization, J. Quant. Spectrosc. Radiat. Transf., vol. 67, no. 5, pp. 327-363, 2000.
- Bohren, C. F. and Huffman, D. R., Absorption and Scattering of Light by Small Particles, New York: Wiley, 1983.
- Born, M. and Wolf, E., Principles of Optics, 7th ed., New York: Cambridge University Press, 1999.
- Coelho, P. J., Bounded skew high order resolution schemes for the discrete ordinates method, J. Comput. Phys., vol. 175, no. 2, pp. 412-437, 2002.
- Dombrovsky, L. A., Radiation Heat Transfer in Disperse Systems, New York: Begell House, 1996.
- Dombrovsky, L., Randrianalisoa, J., Baillis, D., and Pilon, L. Use of Mie theory to analyze experimental data to identify infrared properties of fused quartz containing bubbles, Appl. Opt., vol. 44, no. 33, pp. 7021-7031, 2005.
- Dombrovsky, L., Randrianalisoa, J., and Baillis, D., Modified two-flux approximation for identification of radiative properties of absorbing and scattering media from directional-hemispherical measurements, J. Opt. Soc. Am. A, vol. 23, no. 1, pp. 91-98, 2006.
- Dombrovsky, L. A., Tagne, H. K., Baillis, D., and Gremillard, L., Near-infrared radiative properties of porous zirconia ceramics, Infrared Phys. Technol., vol. 51, no. 1, pp. 44-53, 2007.
- Liou, B.-T. and Wu, C.-Y., Radiative transfer in a multi-layer medium with Fresnel interfaces, Heat Mass Transfer, vol. 32, no. 1-2, pp. 103-107, 1996.
- Modest, M. F., Oblique collimated irradiation of an absorbing, scattering, plane-parallel layer, J. Quant. Spectrosc. Radiat. Transf., vol. 45, no. 5, pp. 309-312, 1991.
- Modest, M. F., Radiative Heat Transfer, 2nd ed., New York: Academic, 2003.
- Muresan, C., Vaillon, R., Menezo, C., and Morlot, R., Discrete ordinates solution of coupled conductive radiative heat transfer in a two-layer slab with Fresnel interfaces subject to diffuse and obliquely collimated irradiation, J. Quant. Spectrosc. Radiat. Transf., vol. 84, no. 4, pp. 551-562, 2004.
- Özişik, M. N., Radiative Transfer and Interaction with Conduction and Convection, New York: Wiley, 1973.
- Sacadura, J.-F. and Baillis, D., Experimental characterization of thermal radiation properties of disperse media, Int. J. Therm. Sci., vol. 41, no. 7, pp. 699-707, 2002.
- Santarelli, F., Stramigioli, C., and Spiga, G., Rigorous and simplified approach to the radiative transfer in an absorbing and anisotropically scattering slab with a reflecting boundary, Int. J. Heat Mass Transfer, vol. 23, no. 6, pp. 853-860, 1980.
- Sobolev, V. V., Light Scattering in Planetary Atmospheres, Moscow: Nauka (in Russian), 1972.
- Sobolev, V. V., Light Scattering in Planetary Atmospheres, Oxford, UK: Pergamon, 1975.
- Spiga, G., Santarelli, F., and Stramigioli, C., Radiative transfer in an absorbing and anisotropically scattering slab with a reflecting boundary, Int. J. Heat Mass Transfer, vol. 23, no. 6, pp. 841-852, 1980.
- Tagne, H. T. K. and Baillis, D., Isotropic scaling limits for one-dimensional radiative heat transfer with collimated incidence, J. Quant. Spectrosc. Radiat. Transf., vol. 93, no. 1-3, pp. 103-113, 2005.
- van de Hulst, H. C., Light Scattering by Small Particles, New York: Wiley, 1957.
- van de Hulst, H. C., Light Scattering by Small Particles, New York: Dover, 1981.
- Wu, C.-Y. and Liou, B.-T., Discrete-ordinate solutions for radiative transfer in a cylindrical enclosure with Fresnel boundaries, Int. J. Heat Mass Transfer, vol. 40, no. 10, pp. 2467-2475, 1997.