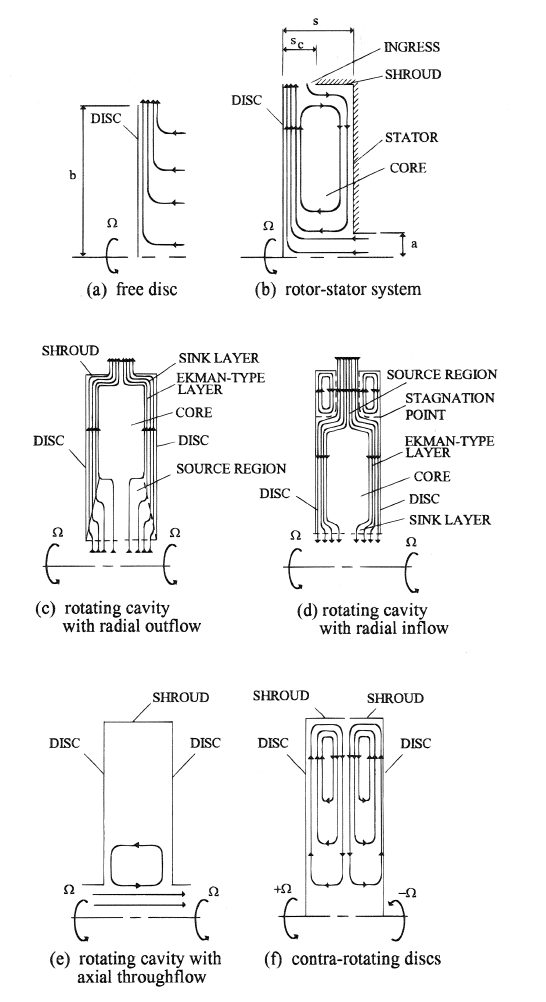
The flow and heat transfer associated with air cooled gas turbine discs can be modeled using simple rotating disc systems, as shown in Figure 1, where one disc rotates close to a second disc, which may be rotating or stationary. It is convenient to classify the system by Γ, the ratio of the speed of the slower disc to that of the faster one: Γ = –1, 0, +1 correspond to contra-rotating discs, a rotor-stator system and corotating discs, respectively.
In rotating flows, Coriolis forces can create phenomena not observed in nonrotating cases. Some of these phenomena are outlined below, and more details are given by Greenspan (1968) and Owen and Rogers (1995).
It is convenient to use cylindrical polar coordinates (r,  , z) rotating with angular speed Ω about the axis r = 0; the velocity components in this rotating frame are u, v and w. It is assumed that the axial clearance, s, between the discs is large enough for separate boundary layers to develop on each disc, between which is a rotating core of fluid.
, z) rotating with angular speed Ω about the axis r = 0; the velocity components in this rotating frame are u, v and w. It is assumed that the axial clearance, s, between the discs is large enough for separate boundary layers to develop on each disc, between which is a rotating core of fluid.
For quasi-solid-body rotation, the linear Coriolis accelerations (2Ωu, 2Ωv) can be much larger than the nonlinear inertial accelerations. If the nonlinear terms are neglected, the Navier-Stokes equations reduce to a system of linear equations. Similarly the boundary-layer equations reduce to a linear system referred to as the Ekman-layer equations [Ekman (1905)], which are written below together with the continuity equation:


If viscous effects are negligible (for example, in the core outside the boundary layers on the discs), the Taylor-Proudman theorem [see Greenspan (1968) or Owen and Rogers (1995)] produces some important results. In particular, for axisymmetric flow in the rotating core it follows from Eqnations (1) to (4) that
where the subscript ∞ denotes that z → ∞. That is, for an inviscid fluid in quasi-solid-body rotation, the flow has a two dimensional tendency: all three components of velocity are invariant with z and, for axisymmetric flow, the radial component is zero.
This two-dimensional tendency makes the boundary layer on a rotating disc resistant to separation. For example, a step change in the surface of a stationary disc would cause boundary-layer separation, but if the disc were rotating in a rotating fluid then the flow could remain attached to the step.
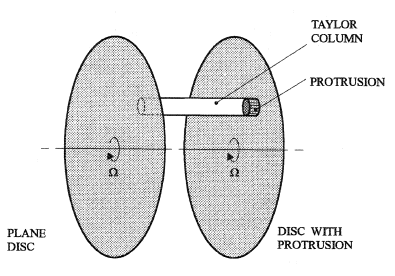
Consider the case of solid-body rotation between two discs, on one of which is a protrusion, where the speed of the discs is suddenly changed from Ω to Ω' . Initially, the speed of the core of fluid is different from that of the discs and fluid flows around the protrusion. However the two-dimensional tendency causes the effect of the protrusion to extend axially throughout the fluid creating a so-called transverse Taylor column, as shown in Figure 2. The fluid flows around this column as though it were a solid cylinder!
A longitudinal Taylor column occurs when a small object is moved axially across a fluid in solid body rotation. A cylinder of fluid is formed, part of which is pushed ahead of the object and part is pulled behind. The rest of the fluid is unaffected by this motion.
Although the two-dimensional tendency arises from an inviscid fluid in solid-body rotation, it has been observed in a variety of rotating-disc systems even when the flow in the boundary layers on the discs is turbulent. In fact the very structure of turbulence in a rotating fluid is affected by this tendency.
Another characteristic of inviscid rotating flows is cyclonic and anti-cyclonic circulation. These nonaxisymmetric circulations often occur in rotating fluids in which buoyancy effects are significant. A cyclonic vortex, which rotates in the same sense as the rotating discs, is associated with low pressure at its center; an anti-cyclonic vortex is associated with high pressure. A pair, or pairs, of these vortices create nonaxisymmetric variations in pressure that provide the necessary Coriolis forces to allow radial inflow and outflow of fluid in the rotating core.
For axisymmetric, incompressible flow, the linear Eqnations (1) to (4) can be solved to give
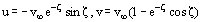
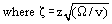
ν being the kinematic viscosity of the fluid and the subscript ∞ referring to conditions in the core outside the boundary layers; the latter are referred to as Ekman layers. The solutions for u and v are oscillatory, u becoming zero when ζ = nπ, n being an integer, and the thickness of the Ekman layer is taken to correspond to n = 1, where ζ = π and z =  . That is, the Ekman layer thickness is invariant with radius. A point to note is that u and v∞ are of opposite sign: if the inviscid core rotates faster than the disc (that is, v∞ > 0) then the flow will be radially inward (u < 0) in the Ekman layer, and vice versa.
. That is, the Ekman layer thickness is invariant with radius. A point to note is that u and v∞ are of opposite sign: if the inviscid core rotates faster than the disc (that is, v∞ > 0) then the flow will be radially inward (u < 0) in the Ekman layer, and vice versa.
An important special case is the rotating cavity (Γ = 1) where there is a superposed radial inflow or outflow of fluid between the discs and where the flow is symmetrical about the midplane (z =  ). According to Equation (5), ∂w∞/∂z = 0, and symmetry implies that w∞ = 0 in the rotating core. In this case, the Ekman layers are therefore nonentraining boundary layers.
). According to Equation (5), ∂w∞/∂z = 0, and symmetry implies that w∞ = 0 in the rotating core. In this case, the Ekman layers are therefore nonentraining boundary layers.
Although the above results are associated with linear, laminar Ekman layers, similar phenomena have been observed for turbulent flow and for cases where the linear assumptions are not strictly valid. Such boundary layers are often referred to as Ekman-type layers.
For rotating-disc systems, there is a strong similarity between the energy equation and the tangential momentum equation [see Owen and Rogers (1989)]. For the case of a single disc rotating in a quiescent fluid (the so-called "free disc"), the analogy is exact if the Prandtl Number is unity and the surface temperature of the disc increases quadratically with radius. The latter condition implies that
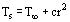
where the subscripts s and ∞ refer to the surface of the disc and the inviscid fluid, respectively, and c is a constant. Under these conditions,

where Nuav is the radially-weighted average Nusselt Number, Reφ is the rotational Reynolds Number and Cm is the moment coefficient based on the frictional moment on the disc.
It also follows for this special case that the adiabatic-disc temperature, Ts,ad is given by
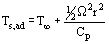
The driving potential for heat transfer is Ts – Ts,ad and the heat flux tends to zero as Ts tends to Ts,ad. For practical applications, it is difficult to determine Ts,ad and most experimentalists use a reference temperature that is convenient to measure, such as the temperature of the fluid at inlet to the system. This can often result in measured Nusselt numbers becoming negative, particularly when the surface temperature of the disc decreases with radius. Whilst the fluid may be colder than the disc at inlet to the system, there may be a radius beyond which the disc is colder than the fluid: the heat flux then becomes negative even though the reference temperature difference remains positive.
REFERENCES
Ekman, V. W. (1905) On the influence of the earth's rotation on ocean-currents, Ark. Mat. Astr. Fys., 2, 1–52.
Greenspan, H. P. (1968) The Theory of Rotating Fluids, Cambridge University Press, London.
Owen, J. M. and Rogers, R. H. (1989) Flow and Heat Transfer in Rotating Disc Systems, Vol. 1: Rotor-stator systems. Research Studies Press, Taunton, John Wiley, New York.
Owen, J. M. and Rogers, R. H. (1995) Flow and Heat Transfer in Rotating Disc Systems, Vol. 2: Rotating cavities, Research Studies Press, Taunton, John Wiley, New York.
References
- Ekman, V. W. (1905) On the influence of the earth's rotation on ocean-currents, Ark. Mat. Astr. Fys., 2, 1–52.
- Greenspan, H. P. (1968) The Theory of Rotating Fluids, Cambridge University Press, London.
- Owen, J. M. and Rogers, R. H. (1989) Flow and Heat Transfer in Rotating Disc Systems, Vol. 1: Rotor-stator systems. Research Studies Press, Taunton, John Wiley, New York.
- Owen, J. M. and Rogers, R. H. (1995) Flow and Heat Transfer in Rotating Disc Systems, Vol. 2: Rotating cavities, Research Studies Press, Taunton, John Wiley, New York.